William is eighteen years old, and he excels in drama and art. In addition, he’s an avid reader and writes poems and short stories in his spare time. However, he cannot graduate with his senior class because he doesn’t have the required credits in mathematics.
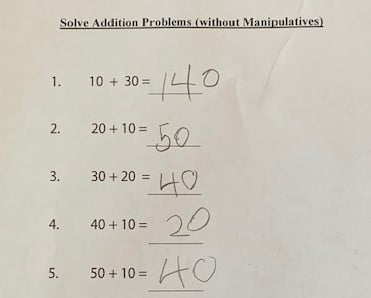
Although he has average intelligence, William has consistently done poorly in mathematics since primary school. After intensive tutoring and years of practice, he has finally become somewhat competent at basic facts and operations, but he has yet to learn how or when to apply them. When taking a math test, he takes numbers from each problem and inserts them into the algorithms he has memorized when studying for the test.
Like William, many people have problems learning mathematics. The nature of their difficulties varies. Some students can master basic facts but can’t do higher mathematics; some can do higher math but can’t master the basics; some can follow math procedures one day but cannot follow them the next day. Others may perform mathematical algorithms well in one situation but can’t apply them to new situations. Math disabilities can be very frustrating due to the complexity and variety of problems.
Table of contents:
- What is dyscalculia?
- What are the types of dyscalculia?
- What are the different subtypes of dyslexia?
- Key takeaways
What is dyscalculia?
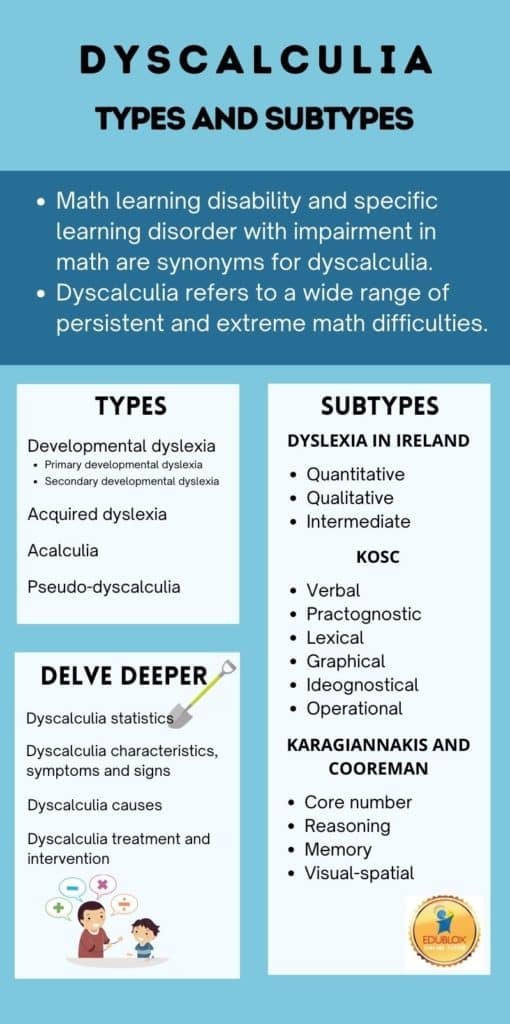
The terms math learning disability and specific learning disorder with impairment in math are synonyms for dyscalculia.
Dyscalculia refers to a wide range of persistent and extreme difficulties in math, including weaknesses in understanding the meaning of numbers and difficulty applying mathematical principles to solve problems. It is the most widely used term for disabilities in arithmetic and mathematics.
Coined in the mid-20th century, the word dyscalculia has both Greek and Latin origins: the Greek prefix ‘dys’ means ‘badly,’ while ‘calculia,’ from the Latin ‘calculare’ means to count. Literally, dyscalculia means to count badly; the reality is much more complex.
What are the types of dyscalculia?
Developmental dyscalculia is used to distinguish the problem in children and youth from similar problems experienced by adults after severe head injuries or as a result of a stroke. This type of dyscalculia is known as acquired dyscalculia or acalculia.
Developmental dyscalculia is a difficulty acquiring basic arithmetic skills not explained by inadequate schooling, emotional instability, or intellectual disability (IQ below 70). Children with developmental dyslexia may perform well in non-numerical academic subjects and have superior intelligence.
Some researchers propose that the scientific community differentiate between primary and secondary developmental dyscalculia.
- Primary developmental dyscalculia is characterized by a severe deficit in numerical or arithmetic functioning caused by different underlying biological factors.
- Secondary developmental dyscalculia denotes individuals whose impaired numerical capacity can be explained entirely by non-numerical impairments, such as attention or working-memory processes (Kaufmann et al., 2013; Price and Ansari, 2013).
Students with pseudo-dyscalculia have severe math anxiety and may even develop math phobia (arithmophobia). Those who have been supported to overcome their math anxiety will potentially be able to function very well in math. However, because of their difficulties with math, most dyscalculics have some anxiety about math (Hornigold, 2015).
What are the different subtypes of dyscalculia?
According to the website Dyslexia in Ireland, dyscalculia can be broken down into three subtypes:
- Quantitative dyscalculia is a deficit in the skills of counting and calculating.
. - Qualitative dyscalculia results from difficulties in comprehension of instructions or the failure to master the skills required for an operation.
. - Intermediate dyscalculia involves the inability to operate with symbols or numbers.
.
Based on his experience with arithmetic learning problems, Kosc (1974) described six subtypes:
- Verbal dyscalculia refers to problems in naming the number of things.
. - Practognostic dyscalculia refers to problems in manipulating things mathematically — for example, comparing objects to determine which is larger.
. - Lexical dyscalculia refers to problems in reading mathematical symbols, including operation signs (+, – ) and numerals.
. - Graphical dyscalculia refers to problems in writing mathematical symbols and numerals.
. - Ideognostical dyscalculia refers to problems in understanding mathematical concepts and relationships.
. - Operational dyscalculia refers to problems in performing arithmetic operations.
.
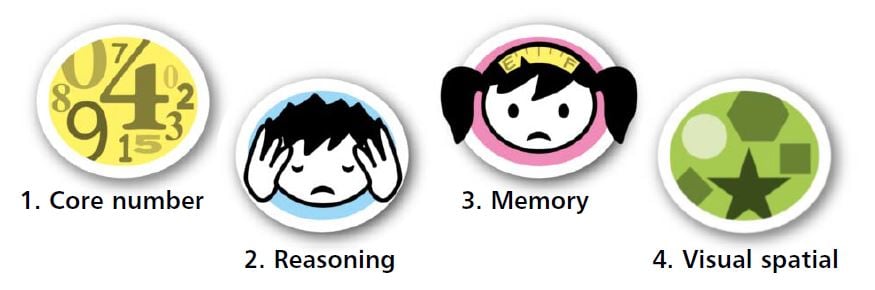
Based on the cognitive difficulties that underpin dyscalculia, Karagiannakis and Cooreman (2015) identified four subtypes:
- Core number
- Reasoning
- Memory
- Visual-spatial
Dyscalculic students may have difficulty in all or maybe just one or two of these areas.
.
.
These subtypes of dyscalculia have not been independently verified, and they are quite difficult to differentiate in students with arithmetic learning disabilities. Nevertheless, these discussions illustrate students’ many problems in arithmetic and mathematics.
Edublox offers live online tutoring to students with dyscalculia. Our students are in the United States, Canada, Australia, and elsewhere. Book a free consultation to discuss your child’s math learning needs.
.
Key takeaways
Delve deeper
Dyscalculia statistics
There is no general agreement on the precise meaning of the term dyscalculia. Therefore, reports of dyscalculia’s prevalence vary depending on the definition and situation. Research suggests it has the same prevalence as dyslexia (about 6–8% of children), although it is far less widely recognized by parents and educators.
Dyscalculia characteristics, symptoms, and signs
The most generally agreed-upon feature of children with dyscalculia is difficulty learning and remembering arithmetic facts. The second feature of children with dyscalculia is difficulty executing calculation procedures, with immature problem-solving strategies, long solution times, and high error rates. There are many other characteristics, symptoms, and signs.
What causes dyscalculia?
Most problems can only be solved if one knows what causes that particular problem. A viable point of departure would thus be to ask: what causes dyscalculia? We investigate genetic influences, cognitive deficits, mathematical skills, and brain differences.
Dyscalculia treatment and intervention
We discuss the most important strategies that need to be applied to help a student overcome a math learning disability. We also look at the Edublox program that offers online help to students with mild to severe dyscalculia.
Authored by Susan du Plessis (B.A. Hons Psychology; B.D.), an educational specialist with 30+ years’ experience in the learning disabilities field.
Medically reviewed by Dr. Zelda Strydom (MBChB).
.
